Abstract
Response and toxicity prediction is essential to rational implementation of cancer therapy. The biologic effects of radionuclide therapy are mediated via a well-defined physical quantity, the absorbed dose, which is defined as the energy absorbed per unit mass of tissue. The concepts, basic definitions, and different approaches to the clinical implementation of absorbed dose estimation are reviewed in this article. Ongoing efforts to improve the accuracy of dosimetry calculations are discussed, as well as studies examining the relationship between absorbed dose and response. Particular attention is placed on the marrow and kidney as dose-limiting organs. Finally, the potential role of radiobiologic modeling in helping to account for differences in dose rate and spatial distribution are reviewed. A treatment planning approach to radionuclide therapy will eventually require incorporation of biologic and radiobiologic considerations. Until such methods are developed and validated, absorbed dose remains an important variable—but still one of several— likely to predict response in an individual patient.
Response and toxicity prediction is essential to the rational implementation of cancer therapy. Unlike most other systemic treatments, the biologic effects of radionuclide therapy are mediated via a well-defined physical quantity, the absorbed dose, which is defined as the energy absorbed per unit mass of tissue. Long- and well-established cancer treatment experience in radiotherapy has provided ample evidence that absorbed dose may be used to predict biologic response (1).
To state that absorbed dose alone would predict response is an oversimplification, however. As has been recognized in radiotherapy and is beginning to be appreciated in radionuclide therapy, the rate at which the absorbed dose is delivered, the manner by which it is delivered (β-particles, α-particles, or Auger electrons), the radiobiologic characteristics of the tumor or normal organ, and the treatment history of the patient all affect response to a specific total absorbed dose (2).
The concepts, basic definitions, and different approaches to the clinical implementation of absorbed dose estimation are reviewed in this article. Current efforts to improve absorbed dose estimates by using more realistic anatomic models and implementing radiobiologic models that can be used to account for dose rate and uniformity are also reviewed. Throughout this review, response is defined as an acute, deterministic response, such as toxicity or tumor shrinkage, rather than a stochastic or carcinogenic effect, such as leukemia.
ABSORBED DOSE DEFINED
It is important at the outset to make a distinction between the terms “absorbed dose” and “dose.” The term “dose” does not have a precise meaning in radionuclide dosimetry. In practice, this term has been used to describe administered activity as well as absorbed dose. In most cases, the distinction can be made on the basis of context. The appropriate term for the quantity of interest in dosimetry, however, is absorbed dose (D), expressed in units of Gray (“rad” in older texts; 1 Gy = 100 rad). This is defined as the energy (E) absorbed in a particular mass of tissue, divided by the tissue mass (M):
 Eq. 1 In radionuclide therapy:
Eq. 1 In radionuclide therapy:
 Eq. 2
Eq. 2 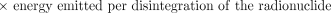

Cumulated Activity
The first term in the expression for E depends on the half-life of the radionuclide and its spatial and temporal distribution. The latter are typically obtained by imaging or sampling. In a typical clinical scenario, images collected at different times after injection of the radiopharmaceutical are used to estimate the amount or concentration of radioactivity in a specific region. The level of activity obtained at different times after injection, plotted against time, gives a time-activity curve for a particular organ. The integral of this curve gives the total number of disintegrations or the cumulated activity (Ã) for the region. This is illustrated in Figure 1. In general, the cumulated activity must be determined for each patient.
Sample time-activity curve for the liver (LI) is depicted. Open circles represent measured time points. Solid line is monoexponential fit to data given by expression A(t), where A0 is injected activity, λeff is effective clearance rate given by (Ln(2)/Tp + Ln(2)/Tb), and fLI is fraction of administered activity in liver, back-extrapolated to injection time. Integral of expression from t = 0 to infinity gives expression shown in box. Residence time (τ) may be derived as shown in second box.
Energy Emitted Per Disintegration
The next term in equation 2 is the total energy emitted per disintegration (Δ) of the radionuclide. This is a property of the radionuclide and is independent of all other factors involved in estimating absorbed dose. It may be obtained from standard physics or dosimetry tables (3,4). The total energy emitted is usually tabulated according to emission type (e.g., photon, β-particle, Auger electron, or α-particle).
Absorbed Fraction
The last term in equation 2 accounts for the emission type, energy, and also the geometry and characteristics of the source and target tissue to provide a net factor that converts the total energy emitted in a particular source region to that absorbed in the region (i.e., self-dose) or in other regions (i.e., cross-organ dose). This absorbed fraction factor (φ) is generally determined by Monte Carlo calculation (5,6).
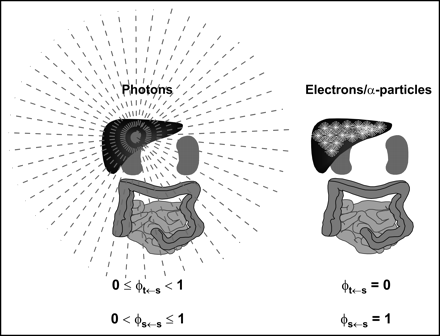
The spectrum of emission types will determine the fraction of energy emitted by a radionuclide that is absorbed by a particular target mass. Radionuclide emissions may be broadly categorized according to their absorption properties. Particulate emissions, such as β- or α-particles are generally absorbed within the tissue of origin. Photons, depending on their energy, will deposit energy in both the source tissue and other adjacent and nonadjacent tissues (Fig. 2).
Different tissue absorption properties of photons versus electron or α-particle emissions of radionuclide are illustrated. Photons originating in liver, for example, depending on energy, can irradiate distant organs. Correspondingly, not all of photon energy emitted within source organ will be absorbed by source organ, as reflected in possible range of absorbed fraction values for photons. In contrast, great majority of electron (Auger and β-particles) or α-particle energy will be absorbed very close to emission source and within source tissue. Correspondingly, energy absorption to other tissues is negligible.
MIRD COMMITTEE SCHEMA
Once the numerator of equation 1 has been obtained (i.e., the energy absorbed in a target mass), it is divided by the mass of the target to yield the absorbed dose to the target. Symbolically, the equation is expressed as follows:
 Eq. 3 where ÃS = cumulated activity in source region S; Δ = energy emitted by the radionuclide per disintegration; φT←S = fraction of energy emitted by the radionuclide in source region S that is absorbed in the target region, T; and MT = mass of region T.
Eq. 3 where ÃS = cumulated activity in source region S; Δ = energy emitted by the radionuclide per disintegration; φT←S = fraction of energy emitted by the radionuclide in source region S that is absorbed in the target region, T; and MT = mass of region T.
This general equation is the starting point for most current approaches to absorbed dose estimation. The equation describes the dose contribution to a target region from a single region.
The derivation as well as the conceptual framework used to arrive at this expression is attributed to the early work of the MIRD Committee, which also established the most commonly used practical approach for estimating absorbed dose. This was accomplished by isolating and tabulating values for those factors of the absorbed dose calculation that can be generalized. As illustrated in Figure 3, the MIRD Committee reduced equation 3 into a product of 2 values, the cumulated activity in a source region and S, the absorbed dose to a target region per unit cumulated activity in the sources (7). The total absorbed dose (DT) to a target region (T) is then the sum of dose contributions to the target from different source regions:
 Eq. 4
Eq. 4
MIRD schema is illustrated. Terms in absorbed dose equation that are independent of radionuclide biodistribution have been compiled and tabulated as S values. S values are tabulated for individual radionuclides according to source-target region pairs. When source-target regions are individual organs or subregions of organs, fixed geometry models are required to establish geometry, relative orientation, composition, and mass of different source-target organs or organ subregions.
The absorbed fractions (φT←S) required to obtain S values were calculated by generating an idealized model of human anatomy defined as a collection of appropriately placed distinct organ volumes with mass and composition that were selected to reflect a typical or standard human anatomy (i.e., standard man), later extended to include female and pediatric models (8). For each radionuclide, a certain number of decays were uniformly distributed throughout each idealized organ volume, and the absorbed fraction from each source-target organ pair was calculated by Monte Carlo simulation. The original calculations were performed in the late 1970s, and, given the limited computational power available at the time, several simplifying assumptions were made to perform these calculations (6). Application of the resulting S values, therefore, to patient anatomies that deviate substantially from the idealized model will lead to errors. Furthermore, use of a standard model precluded the tabulation of S values for tumors, because tumors do not come in standard dimensions or positions. In diagnostic nuclear medicine, this has not been a concern, because the absorbed doses to normal organs are of interest and also because the administered activities generally used are well below levels that can result in toxicity. In therapeutic nuclear medicine, however, the absorbed dose to tumors is important in evaluating treatment efficacy. Accurate estimation of the absorbed dose to normal organs is also important in assessing likely toxicity. The dosimetry requirements of therapeutic nuclear medicine have led to ongoing interest and improvements in radionuclide dosimetry (9). Notable advances are briefly summarized in the following sections.
Extensions to Idealized Phantom Geometries
Since publication of the initial S value table by the MIRD Committee, additional S values have been generated to facilitate absorbed dose estimation for anatomies and dose distributions that differ from the original anthropomorphic phantom. In addition to female and pediatric S value tables, S values have also been generated for organ subregions and for cellular dimensions (10–16,17). The latter make it possible to estimate the mean absorbed dose to the nuclear or whole cell volume from a uniform distribution of activity in different cellular compartments (e.g., cell surface, cytoplasm). These extensions reflect the generality of the MIRD Committee formalism. To support the calculation of nonuniform absorbed doses and to account for nonuniform activity distributions at the level of imaging instrumentation voxels, the MIRD Committee has also published S value tabulations for different voxel sizes and source-target voxel distances (18). The resulting S value tabulations facilitate absorbed dose calculations by separating potentially lengthy and complex Monte Carlo calculations from the task of estimating absorbed dose. Because use of previously tabulated S values requires a fixed anatomic model, this approach is not easily amenable to geometries that deviate substantially from the fixed anatomic models. Voxel S values overcome this problem and have been adopted in several dose calculation programs (19–21).
Fixed Geometry-Based Dosimetry Software
Several software packages have been developed to implement the S value methodology. The most widely-used of these, MIRDOSE3, incorporates S factors for 223 radionuclides and 10 different anthropomorphic models, including standard male, female, and pediatric geometries (22). This software is no longer being distributed and has been replaced by Organ Level Internal Dose Assessment (OLINDA; Vanderbilt University). OLINDA is U.S. Food and Drug Administration (FDA) approved as a device and includes S values specific to 10 phantoms and 5 organ models for more than 800 radionuclides, including α-particle emitters, which were not previously included in S value tabulations. The program also includes a pharmacokinetic module that may be used to determine organ cumulated activities.
MABDOSE (University of Colorado), another package that also implements fixed geometry, allows the user to place spherically shaped tumors within the simplified anatomic model originally described by the MIRD Committee. To do this, an on-the-fly Monte Carlo simulation was incorporated into the code (23,24). This method could accommodate tumor dosimetry within the idealized geometry defined by the MIRD Committee.
Tumor Dosimetry
As noted previously, fixed anatomic models are not easily amenable to tumor dosimetry. Several approaches have been developed for estimating absorbed dose to tumors and the dose contribution from tumors to normal organs. The simplest approximation is made by assuming that all electrons are deposited locally and that the relative contribution to the tumor absorbed dose from photons is negligible. Alternatively, the fraction of electron energy absorbed may be considered, assuming the tumor can be modeled as a sphere (4,5). This is an important correction for tumors with diameters in the range of the path length of electron emissions. Depending on the radionuclide, this correction should be made for tumors with diameters <0.5–1 cm. The photon contribution to larger tumors becomes relevant as tumor diameter increases. Using tables of photon absorbed fraction to spheres or ellipsoids, the photon self-dose may be added by assuming that the tumor is a sphere or ellipsoid (6). If this assumption is made, the photon dose to and from normal organs may also be calculated by placing the idealized tumor geometry in a defined position relative to the standard geometry used for the S factor calculations. If a point-kernel convolution technique is used in estimating absorbed dose, the true tumor and normal tissue geometry as well as the activity distribution may be considered to yield a spatial absorbed dose or dose-rate distribution (20,21,25–31). Tissue composition and density variations are not easily accounted for using point-kernel techniques. To account for these, Monte Carlo techniques are needed to estimate absorbed dose (32–39).
THREE-DIMENSIONAL (3D) IMAGING-BASED DOSIMETRY
The increase in computer processing power and the availability of 3D imaging methodologies, particularly with SPECT/CT and PET/CT, have provided an impetus for direct image-based dosimetry techniques. The essential requirements for 3D imaging-based dosimetry are (a) the availability of 3D anatomic imaging studies such as CT or MRI; (b) at least 1 3D imaging study of the radioactivity distribution (e.g., PET or SPECT); and (c) software that implements a point-kernel or Monte Carlo calculation methodology to estimate the spatial distribution of absorbed dose. To define the anatomy and also provide tissue density information, a CT scan over the region of interest is required. To provide the activity distribution, a PET or SPECT scan is required. If absorbed dose rather than absorbed dose rate is desired, then kinetic information is required. In conventional dosimetry, kinetic information that is averaged over the whole source organ volume suffices. In 3D dosimetry, however, variations in pharmacokinetics within an organ must be considered. Ideally, kinetic information on a voxel-by-voxel basis should be used. This would require multiple SPECT or PET studies taken over time, each registered to the other as well as to the CT study. Assuming such data are available, a voxel-by-voxel-based integration of the activity would have to be performed to yield a 3D representation of residence time or cumulated activity. Alternatively, it is possible to derive a residence time or cumulated activity image by using planar imaging to obtain kinetics and SPECT or PET to obtain spatial distribution. This is a compromise and represents an approximation to the actual cumulated activity, because different organs (or tissues) with different kinetics may be superimposed on planar views. The residence time or cumulated activity image may be obtained by assuming that all points making up the spatial distribution of activity follow the same kinetics. The mean dose over a target volume is determined by taking the mean of all values in the dose array. Either integral or differential dose-volume histograms can be generated. In the former, the percentage of volume receiving less than or equal to a particular dose value is plotted as a function of the dose value. In the latter, the percentage of volume receiving a particular absorbed dose range is plotted versus the dose range. The dose distribution can also be displayed as an image or as isodose levels superimposed on anatomic or radioactivity images.
3D Imaging-Based Dosimetry Software
Many researchers have pursued and contributed to 3D imaging-based patient-specific dosimetry (21,23,29–31,33–36,39). This section will focus on the 2 most fully developed packages, 3D Internal Dosimetry (3D-ID) and DOSIMG (Lund University, Lund, Sweden) (33,40).
The 3D-ID software package takes the distribution of radiolabeled antibody for a given patient (from SPECT or PET) and combines it with anatomic information (from CT or MRI) to yield absorbed dose estimates that are specific to a particular patient’s biodistribution and anatomy (25,30,41–43). This work introduced the concept of dose-volume histograms for internally administered radionuclides (44). The 3D-ID software package may be used to perform both Monte Carlo and point-kernel-based calculations. It has been used to examine the impact of different radionuclides on dose distribution, given a fixed cumulated activity distribution (34). 3D-ID has been used to perform a detailed analysis of tumor dose versus response in the treatment of non-Hodgkin’s lymphoma (NHL) using 131I-anti-B1 antibody (42). The point-kernel module in 3D-ID and data from a clinical trial of 131I-labeled anti-B1 antibody were used. The analysis did not reveal a statistically significant dose-response relationship. Using a more robust and validated SPECT quantitation methodology in patients who had not been treated previously, Koral et al. (45) were able to demonstrate a dose-response relationship. This was observed only in a selected subset of 15 (pelvic or abdominal) tumors (identified on CT and <10 cm3 in size) out of 43 in a subset of an already selected patient population (partial responders). More recently, 3D-ID has been used in thyroid cancer patients with 124I PET data and CT (43). This study demonstrated the use of multiple PET image studies registered across time and integrated, voxel by voxel, to provide a 3D cumulated activity image used in the dosimetry calculation.
The DOSIMG software has been used with mathematical anthropomorphic phantoms to examine the impact of different quantitative SPECT algorithms on Monte Carlo-derived absorbed dose calculations. Mathematical phantom-derived CT and SPECT images were generated, and dose calculations derived from these were compared with “true” dose results derived from the actual mathematical phantom data (46). The SPECT quantitation methodologies derived from this work were subsequently applied to 111In/90Y dosimetry (47).
RED MARROW DOSIMETRY
In the majority of nonmyeloablative radionuclide therapy, red marrow toxicity is dose limiting. As a result, the red marrow has received the greatest attention in terms of developing and examining dosimetry methodologies (48–55). The dosimetry approaches may be broadly categorized as blood based or imaging based, depending on whether or not the radiopharmaceutical specifically localizes to blood, bone, or marrow components, including tumor micrometastases in the marrow. A comprehensive review and recommendations for performing marrow dosimetry have been published in the case that the radiopharmaceutical binds to blood, bone, or marrow components (51). If this does not occur, then blood-based methods that relate the activity concentration of the radiopharmaceutical in blood or plasma to the concentration in the red marrow have been described (48,49). It is important to note that these methods are largely specific to intact radiolabeled antibodies. In particular, by assuming rapid equilibration of radiolabeled antibodies in the plasma and extracellular fluid of the red marrow, a factor of 0.3–0.4 may be derived that relates the concentration of radioactivity in the red marrow to that in the blood (the red marrow-to-blood ratio). In murine studies, this range of concentration ratios has been confirmed experimentally for intact, 150-kD antibodies but not for lower molecular weight fragments that yield a red marrow-to-blood concentration ratio closer to 1 (53).
DOSE-RESPONSE RELATIONSHIPS
The raison d’être for internal emitter dosimetry is to predict response and toxicity. In part, because radionuclide therapy (with the exception of radioiodine treatment for thyroid disease) is a relatively new field, few human studies have been reported that specifically address this question. As a result, the dose-response experience of external beam radiotherapy is used to evaluate potential tumor response or normal organ toxicity. The few reports specifically examining dose-response relationships in humans are briefly reviewed.
Tumor
Because radioimmunotherapy of NHL has resulted in measurable responses, several studies have examined tumor response versus absorbed dose in NHL. In addition to the 2 anti-CD20 antibody reports cited previously, the dose-response relationship in NHL for an anti-CD22 antibody labeled with 90Y has been examined, and no relationship between tumor response (assessed as the percentage change in the sum of perpendicular diameters) and absorbed dose (determined by planar imaging of 111In and using MIRDOSE3) was observed (42,45,56). The authors concluded that this can be partly attributed to the biologic activity of the unlabeled antibody as well as other biologic factors affecting treatment response, as suggested by recent findings of genetic correlates with treatment response to rituximab (57). It is important to note that different dosimetry methodologies were used for these 3 NHL dose-response studies, and the methodology used to estimate absorbed doses will affect dose-response correlations. In solid tumor targeting, a recent review of reported biodistribution, dosimetry, and outcome in radiolabeled CC49 antibody trials found that the reported tumor absorbed-dose estimates were more variable than normal organ absorbed-dose estimates and also found a wide variability in reported dosimetric parameters and methodologies (58). The observation was made that standardization and improved dosimetry may aid in establishing dose-response relationships. In addition to the dosimetry calculation methodology, standardization of data acquisition is also important. A MIRD pamphlet is available that outlines a standard approach to data acquisition (59).
In radiopeptide therapy, wherein substantially higher tumor absorbed doses may be delivered (because marrow toxicity is less limiting as a result of rapid clearance of the radiopeptide from the circulation), a robust tumor dose-response relationship has been observed (60).
Red Marrow
The red marrow is a complex, distributed organ and presents challenges both in terms of estimating activity concentration kinetics for cumulated activity determination and also for Monte Carlo calculation of absorbed fractions.
In estimating the cumulated activity, progress has been made toward adopting a standardized, blood-based approach, and several red marrow dose-response studies have been reported (61–67). One such study found that absorbed dose to the red marrow or total body predicted hematologic toxicity better than administered activity or administered activity per meter squared (67). The analysis and conclusions were confined to 131I-labeled antibodies and antibody fragments that did not bind to blood, bone, or marrow cellular components. Consistent with this observation and based on toxicity correlations in phase I/II trials, the 131I-labeled anti-CD20 antibody, tositumomab (Bexxar; GlaxoSmithKline), which was recently approved by the FDA for treatment of NHL, is prescribed to patients according to total body absorbed dose (68,69). Absorbed dose versus toxicity studies for another FDA-approved anti-CD20 antibody labeled with 90Y, ibritumomab tiuxetan (Zevalin; Biogen Idec), did not show a correlation of absorbed dose with toxicity. As a result, this agent is prescribed on a per-body-weight basis (70,71). The different dose-response observations and prescription schemes have been explained primarily by the difference in half-lives and retention properties of the 2 radionuclides, 131I and 90Y. 131I-labeled antibody is subject to dehalogenation and urinary excretion as free 131I. Given, the 8-d half-life of 131I, interpatient variation in dehalogenation and urinary excretion will substantially affect the whole body and marrow absorbed dose. In contrast, the shorter 2.7-d half-life of 90Y, combined with its chelate conjugation to antibodies, is subject to reduced urinary excretion as free 90Y. In addition to the reduced excretion rate of 90Y-labeled anti-CD20 antibody, the requirement that 90Y kinetics be derived from 111In imaging could also provide a plausible explanation for the absence of a dose-response relationship in this case. The surrogate, 111In, is used for 90Y because 90Y does not emit photons that are easily imaged. It is a pure β-emitter and only bremsstrahlung photons are emitted. In this case, prior treatment and bone marrow reserve have been shown to be better predictors of marrow toxicity. Because patients undergoing radioimmunotherapy have been previously exposed to chemotherapy, the impact of such prior therapy on bone marrow reserve is important in evaluating and interpreting hematopoietic dose-response studies. In this regard, plasma levels of FLT3-L, a cytokine involved in regulating hematopoietic recovery by stimulating hematopoietic proliferation after chemotherapy, has been found useful as a predictor of marrow radiosensitivity that can be used to adjust the anticipated biologic effect of a specific red marrow absorbed dose (72).
All red marrow dosimetry performed to date has relied on a highly stylized representation of the marrow. More detailed representations have recently been developed and are being used to perform Monte Carlo calculations for generating more accurate S values (73–83).
Kidney
Recent instances of irreversible renal toxicity have highlighted the kidney as a possible dose-limiting organ (84–89). The concern over renal toxicity reflects several developments in therapeutic nuclear medicine. Although the developments are highly diverse (encompassing, for example, peptide-mediated receptor targeting, metabolic targeting using bone-seeking radionuclides, and engineered low-molecular-weight multivalent targeting), these can all be generally described as a shift to constructs that have molecular weight substantially lower than the 150,000 Da of intact antibodies (86,88,90). These generally clear from the circulation very rapidly (T1/2 < 1–5 h) and are excreted through the kidneys. Because clearance is so rapid, the red marrow is generally not the dose-limiting organ (assuming no specific uptake in the marrow); instead, renal toxicity becomes a concern. This is best illustrated in the case of peptides that target the somatostatin receptor found on neuroendocrine tumors. The rapid clearance and observed large variations in tumor uptake have required administration of very large levels of radioactivity to deliver effective tumor doses. Clearance through and prolonged retention in the kidneys have resulted in very high kidney absorbed doses that approach or, in fractionated treatment protocols, have exceeded the radiotherapy-derived 23-Gy tolerance limits (which yield nephropathy in 5% of the exposed population in 5 y) (91). As might be expected, the incidence of renal pathology has been highly dependent on the radionuclide used. The Auger-emitter 111In, with particle ranges that are less than a cell diameter, has shown minimal toxicity at estimated kidney absorbed doses of 45 Gy (cumulative administered activities of 58 GBq/m2), whereas 90Y, with long-range emissions of 5 mm in tissue (90% of β-energy deposited in a 5-mm radius sphere), has led to renal toxicity at administered activities in the 1.9 GBq/m2 range (84,92).
Renal toxicity has also been observed in a bone marrow ablation trial using 166Ho-1,4,7,10-tetraazacyclododecane-1,4,7,10-tetramethylene-phosphonic acid in patients with multiple myeloma (88,93). Rapid clearance via the kidneys with minimal kidney retention was observed. The range of estimated kidney absorbed doses was 2.6–14 Gy, well below the 23-Gy tolerance limit (91). Despite this, renal dysfunction was observed in 36% of patients treated, and grade 3 or higher toxicity was observed in 17% of the treated population. Although the treatment protocol included chemotherapy and external radiotherapy, a statistical analysis showed that injected activity per body weight was the only factor with a significant effect on renal toxicity. Radiobiologic analysis of these data has suggested that the very high dose rate resulting from transit of 17–170 GBq 166Ho through the kidneys resulted in absorbed dose rates that translated to biologically effective doses of 40–50 Gy, depending on the radiobiologic parameters used (94,95). These experiences have placed a greater emphasis on improving kidney dosimetry and better understanding of the radiobiologic implications of absorbed dose nonuniformity and delivery rate (15,94–99).
FUTURE DIRECTIONS
As nuclear medicine has grown to include a substantial therapeutic component, the importance of radionuclide dosimetry has also grown. The expectation that radionuclide dosimetry could be used to plan radionuclide therapy in the same way that dosimetry is used to plan external beam radiotherapy has yet to be met. In large part, this unfulfilled expectation is the direct result of the many variables involved in determining the biologic effect of targeted radionuclides. In many but certainly not all instances the most important variables are biologic rather than dosimetric, making radionuclide dosimetry an important—but not the only—determinant of response. To achieve the treatment planning objective in radionuclide therapy, it is important to understand and distinguish between response results dominated by biologic factors versus dosimetry. It is essential, therefore, that ongoing improvements in dosimetry methodology continue so that the role of dosimetry relative to biologic factors is properly evaluated.
Table 1 lists the dosimetric parameters relevant to tumor or normal organ response. Current routinely available dosimetry software provides the first item on the list as a mean absorbed dose averaged over an idealized, spherical tumor or fixed normal organ volume. As indicated previously, efforts to improve on this by providing the spatial distribution of absorbed dose to individual patient anatomy are gaining prominence, and many groups are developing software to accomplish this. An important challenge in these efforts is to provide output that helps in the interpretation of the results. Accurate, detailed absorbed dose calculations are useful only to the extent that they are biologically relevant and easily interpretable.
Dosimetric and Radiobiologic Parameters in Predicting Tumor Response or Normal Organ Toxicity
The uniformity (or lack thereof) of absorbed dose distributions and their biologic implications have been examined intensively, primarily in animal studies (100–108). To address the question of how to best represent the large amount of data in 3D distributions of absorbed dose, it is useful to borrow from the radiotherapy field. One possible approach would be dose-volume histograms to represent dose distributions in targeted radionuclide therapy (44). Another approach is the equivalent uniform dose (EUD) model (104). The EUD model takes this a step farther by introducing the radiobiologic parameters, α and β (the sensitivity per unit dose and per unit dose squared, respectively, in the linear-quadratic dose-response model), to convert the spatially varying absorbed dose distribution into an equivalent uniform absorbed dose value that would yield a biologic response similar to that expected from the original dose distribution.
To date, the rate of absorbed dose delivery has been largely ignored. In addition to the extensive evidence in external radiotherapy, there is also some evidence in radionuclide therapy that absorbed dose rate should not be neglected in trying to predict response. Such preliminary evidence has been cited here for renal toxicity and has also been reported regarding bone marrow toxicity (53). The importance of dose rate will increase as lower molecular weight agents that clear rapidly and that require greater administered activities gain widespread use. In an analogy to the EUD model for evaluating and comparing different spatial distributions of absorbed dose, the biologically effective dose (BED) model may be used to compare the response implications of total absorbed doses delivered at different dose rates. Detailed derivations and reviews of this model and examples of its application to radionuclide therapy have been published (95,109–111). In essence, the approach is similar to that of EUD, in that a particular dose-rate profile is converted to a reference dose rate, based on a radiobiologic model that translates dose-rate distribution to expected response.
Although potentially useful in representing complex spatial and temporal distributions of absorbed dose, such simplification must be implemented with caution, because they rely on the biologic models used and on the model parameters chosen for the calculation. Calculation of EUD requires knowledge of the linear-quadratic model parameters α and β. In addition to these, the BED calculation requires knowledge of μ, the repair rate (assuming exponential repair) for radiation-induced DNA damage. It is important to note that these parameters will be influenced by prior therapy.
The 3 parameters, α, β, and μ, are related to radiosensitivity and, as indicated in Table 1, will be spatially dependent as they are related to hypoxia and proliferation rate. Since these parameters are also highly relevant to response in external radiotherapy, imaging-based measurements are already being investigated (112). As noted, radiosensitivity will also be modulated by exposure to prior therapeutics.
The last item listed in Table 1 relates to the observation that normal organs are hierarchical and that the consequences of radiation damage are highly dependent on the particular group or class of cells that are irradiated and that may arise either by cross-fire from adjacent cells or activity-containing vasculature or by directly concentrating the radiolabeled agent or radionuclide. The “criticality” parameter, therefore, is intended to reflect the likelihood that damage to a particular group of cells will lead to organ failure. Such a parameter is particularly relevant to the kidney, wherein radiation-induced renal failure is generally associated with damage to endothelial cells of the glomerular capillaries (thrombotic microangiopathy) (84,97).
SUMMARY
The biologic effects of radionuclide therapy are mediated via a well-defined physical quantity, the absorbed dose, which is defined as the energy absorbed per unit mass of tissue. This basic formulation, as well as subsequent practical methodologies for estimating absorbed dose, were established by the MIRD Committee, which first published a table of S values, making it possible to convert cumulated activity in different organs to absorbed dose. A fixed geometry model was adopted to calculate S values. S values derived from fixed geometry models have the advantage of not requiring point-kernel or Monte Carlo calculations in estimating absorbed dose. The requirements of therapeutic nuclear medicine have led to ongoing refinements in the fixed geometry models used to derive S values, such that several organ-specific and whole-body age-specific models have been developed and are included in OLINDA, an FDA-approved software package that implements the fixed model approach to absorbed dose calculation. Fixed geometry models have the disadvantage, however, of not matching the actual patient anatomy. This disadvantage is being addressed by developments in 3D imaging-based patient-specific dosimetry software. Examination of whether such new dosimetry methodologies lead to improved dose-response relationships has only recently begun. Such studies are highly dependent on the availability of response data from radionuclide therapy trials. More such data are needed if ongoing improvements in dosimetry methodologies are to be validated against the clinically relevant measure of response prediction. Comparison of dose-response studies from different institutions will require a standardized absorbed dose methodology. Such standardization has been generally achieved for red marrow dosimetry but remains an important problem for normal organ or tumor dosimetry. Recent evidence of renal toxicity at total absorbed doses thought to be safe based on experience in radiotherapy has highlighted the need to examine additional dosimetric parameters such as dose rate and spatial distribution. The translation of such additional data into response probability will require radiobiologic modeling. Such modeling depends critically on the availability of the relevant parameters and on an understanding of how these change after chemotherapy or external beam radiotherapy.
CONCLUSION
In conclusion radionuclide dosimetry is still at an early stage of development. Active research toward developing patient-specific 3D imaging-based dosimetry, coupled with the availability of faster computers, improved Monte Carlo methods, and dual anatomy/radioactivity imaging devices, will improve the physics of absorbed dose estimation. Because these techniques will affect clinical trial expense and patient comfort, the gain from such improvements must be rigorously tested in terms of improved response prediction. A treatment planning approach to radionuclide therapy will eventually require incorporation of biologic and radiobiologic considerations. Efforts in this direction are just beginning. Until such methods are developed and validated, however, absorbed dose remains an important variable—but still one of several—likely to predict response in an individual patient.
Acknowledgments
Support from the National Institutes of Health (grants R01 CA62444, R01 CA 72683, and P01 CA33049) and from the U.S. Department of Energy (grant DEFG02-86ER-60407) are gratefully acknowledged.
Footnotes
Received Aug. 9, 2004; revision accepted Nov. 17, 2004.
For correspondence or reprints contact: George Sgouros, PhD, Department of Radiology, Johns Hopkins University, School of Medicine, 720 Rutland Ave, Baltimore, MD 21205.
E-mail: gsgouros{at}jhmi.edu