Reply: Log Normal Distribution of Cellular Uptake of Radioactivity

REPLY: We thank our colleagues for bringing attention to their article that addressed the impact of nonuniform distributions of radioactivity at the cellular level on cell killing (1). We always strive to refer to all prior literature on a given topic and we sincerely apologize for the omission. We agree that they carefully examined the radiobiologic implications of the distribution of radioactivity at the cellular level and showed that surviving fractions of cells were estimated to be higher than the fractions found with the assumption of uniform uptake. However, we disagree with their contention that they found that their distribution was log normal for the following reasons. To obtain the distribution of radioactivity at the cellular level, Kvinnsland et al. (1) inferred the distribution from a fluorescence intensity distribution acquired on a flow cytometer. As per standard practice in flow cytometry, their data were acquired under logarithmic amplification (Fig. 2 (1)). In their Results, they state that “the distributions of antigen were close to a gaussian-shaped curve on a log scale on the abscissa.” Accordingly, they implemented a logarithmic transformation of their data to enable its use for further analysis (Eq. 8 (1)). It is important to point out that the use of a logarithmic transformation does not necessarily imply that the distribution is log normal. The near-gaussian shape of their distribution on a log scale does suggest that their distribution resembles a log normal distribution. However, they neither made statements nor provided mathematic analyses to indicate the log normal resemblance of their distribution. This may be partly why our literature searches failed to identify their article. We did note in our Discussion that log normal distributions are likely the norm and that most flow cytometry reagents are best visualized under logarithmic amplification (3). With this in mind, it should be noted that other investigators have reported flow cytometry data similar to theirs (2), and we have made similar observations in our unpublished data on the distribution of BrdU antigen in V79 cells.

As pointed out by Kvinnsland et al. in their letter to the editor, we measured the distribution of radioactivity at the cellular level using autoradiographic techniques (3), whereas they infer the distribution from fluorescence intensity measurements obtained with a flow cytometer. We use both techniques in our laboratory and each has its strengths and limitations. The autoradiographic approach is labor-intensive; however, it does actually measure the distribution as opposed to inferring it. Indeed, it is known that the distribution of radioactivity can be significantly different than the distribution of the antibody (4). One criticism of the authors with regard to the autoradiographic approach was that “the distribution has to be measured part by part by varying the concentrations of radiochemicals and exposure times.” Whereas exposure times were varied to obtain track data that cover the entire distribution of cellular activity, concentrations were changed only to examine whether extracellular concentration of radioactivity influenced the shape of the distribution (Fig. 5 (3)). This should be done regardless of the measurement technique. Nevertheless, the authors of the letter raised an excellent question with regard to the potential influence of Poisson statistics on our autoradiographic track distributions and their subsequent analysis. Indeed, if each cell in the population had the same activity, then one would anticipate a Poisson distribution of measured tracks that would change with increasing expectation value (i.e., longer autoradiograph exposure times). With this in mind, the authors point out that our measured distribution may be a convolution of a Poisson distribution and an underlying distribution associated with the radioactivity. We were remiss in not definitively addressing the impact that this may have on our results. To investigate the impact of Poisson statistics on determining the distribution of radioactivity in the cell population from our autoradiographic data, it is necessary to return to the raw data in Figure 3 of Neti and Howell (3). Figures 3A, 3B, and 3C contain track distributions obtained from cell populations that were exposed to 0.52, 3.8, and 67 kBq/mL, respectively (3). The track distributions were acquired from autoradiographs that were developed at different times. Each set of track distribution data includes the number of cells scored with 0–9 tracks per cell as well as the number of cells with an unscoreable number of tracks (>9 tracks). We have examined the effect of Poisson statistics on our analyses of these data both before and after our convolution of the datasets. The data were analyzed with Poisson, log normal, and combined Poisson + log normal distribution functions. The Poisson distribution function is given by P(n) = (cⁿ/n!)e^−c, where n is the number of tracks per cell, c is the expected value <n>, and P(n) is the probability of n discrete tracks per cell. The log normal distribution functions are given in (3). According to Fors et al. (5), the Poisson + log normal compound probability of obtaining a realization n given the mean c and all its possible Poisson realizations k is given by: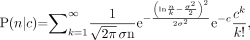where σ is the shape parameter. The capacity of these distributions to describe the various experimental data (t = 0.25, 0.67, 1, 4, 7, 26, and 52 d) were tested by reduced χ² () analyses and compared.

As pointed out by the authors, the Poisson distribution shifts as the mean is increased. However, among the 3 distributions tested, the Poisson distribution gives the highest value for every dataset (poorest fit to the data). The lowest values are obtained with the log normal (t = 0.25, 0.67, 4, 7, 52 d) or Poisson + log normal distribution functions (t = 1, 7 d). A detailed analysis suggests that there is a significant Poisson component in some of the measured track distributions; however, the underlying distribution remains log normal. Notably, the shape parameters (σ) obtained by minimizing are generally within uncertainties with respect to those that were obtained by a least-squares fit of the convolved data to a log normal function (3). It is our intention to publish the details of these analyses elsewhere.

The statistical analyses briefly described here support our conclusion that the distribution of radioactivity in the cell population is well represented by a log normal distribution. As mentioned earlier (3), it is possible that other distribution functions may better explain the experimental data and no attempt was made to ascertain this. We trust that because of the ubiquitous presence of log normal distributions across many fields (6), many investigators in radiation biology may find this distribution useful to fold into their dose–response models. Its implementation is facilitated by several factors. First, and foremost, it is an analytic function that is described by only 2 parameters (σ, μ). Second, the log normal probability density function is provided in standard subroutine libraries (e.g., National Algorithm Group). In closing, we thank Kvinnsland et al. and the editor for providing us with this opportunity to provide further support for the log normal distribution of radioactivity among a cell population.